Stan naprężenia w zginanej belce o zmiennej wysokości –
badania analityczne i numeryczne MES

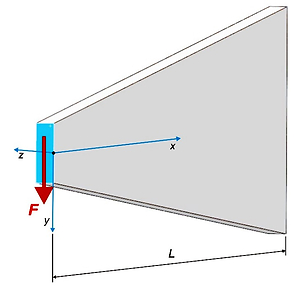
Praca przestawia analizę porównawczą stanu naprężenia w zginanej belce wspornikowej o zmiennej wysokości. Przeprowadzono obliczenia analityczne i numeryczne metodą elementów skończonych. Model analityczny został opisany na podstawie literatury, natomiast model do obliczeń metodą elementów skończonych opracowano z zastosowaniem systemu SolidWorks. Wyniki, tzn. naprężenia wyznaczone analitycznie i numerycznie zostały porównane i zamieszczone w tablicach oraz zilustrowane na rysunkach.

REFERENCJE (14)

Alexandrov A.V., Potapov V.D., Derzhavin B.P., Strength of materials, Moscow, Izd. ”Vyshaya Shkola” 2003. (in Russian).

Google Scholar

Attarnejad R., Semnani S.J., Shahba A., Analysis of non-prismatic Timoshenko beams using basic displacement functions, Advances in Structural Engineering, 14(2): 319-332, 2011.

Google Scholar

Auciello N.M., Ercolano A., A general solution for dynamic response of axially loaded non-uniform Timoshenko beams, International Journal of Solids and Structures, 41(18-19): 4861-4874, 2004.

Google Scholar

Auricchio F., Balduzzi G., Lovadina C., The dimensional reduction approach for 2D nonprismatic beam modelling: A solution based on Hellinger–Reissner principle, International Journal of Solids and Structures, 63: 264-276, 2015.

Google Scholar

Balduzzi G., Aminbaghai M., Sacco E., Füssl J., Eberhardsteiner J., Auricchio F., Non-prismatic beams: A simple and effective Timoshenko-like model, International Journal of Solids and Structures, 90: 236-250, 2016.

Google Scholar

Dado M., Al-Sadder S., A new technique for large deflection analysis of non-prismatic cantilever beams, Mechanics Research Communications, 32(6): 692-703, 2005.

Google Scholar

Gere J.M., Timoshenko S.P., Mechanics of Materials, (Second Edition) Boston, PWS-KENT Pub. Comp. 1984.

Google Scholar

Maalek S., Shear deflections of tapered Timoshenko beams, International Journal of Mechanical Sciences, 46(5): 783-805, 2004.

Google Scholar

Magnucki K., Lewinski J., Analytical modeling of Ibeam as a sandwich structures, Engineering Transactions, 66(4): 357-373, 2018.

Google Scholar

10.

Rajasekaran S., Buckling of fully and partially embedded non-prismatic columns using differential quadrature and differential transformation methods, Structural Engineering and Mechanics, 28(2): 221-238, 2008.

Google Scholar

11.

Rajasekaran S., Free vibration of centrifugally stiffened axially functionally graded tapered Timoshenko beams using differential transformation and quadrature methods, Applied Mathematical Modelling, 37(6): 4440-4463, 2013.

Google Scholar

12.

Shahba A., Attarnejad R., Marvi M.T., Hajilar S., Free vibration and stability analysis of axially functionally graded tapered Timoshenko beams with classical and non-classical boundary conditions, Composites Part B: Engineering, 42(4): 801-808, 2011.

Google Scholar

13.

Slivker V.I., Structural mechanics, Moscow, Pub. Assoc. Structural 2005. (in Russian).

Google Scholar

14.

Zhou D., Cheung K., Vibrations of Tapered Timoshenko Beams in Terms of Static Timoshenko Beam Functions, Journal of Applied Mechanics, 68(4): 596-602, 2001.

Google Scholar

Wyślij swój artykuł

Informacja dla autorów

Archiwum

Udostępnij

Wyślij mailem

ARTYKUŁ POWIĄZANY

Analityczne, numeryczne (MES) i doświadczalne badanie zginania trójwarstwowego pasma płytowego z rdzeniem z pianki metalowej

Badania analityczne, numeryczne MES i doświadczalne wytrzymałości osi pojazdu szynowego

Indeksy

Indeks słów kluczowych

Indeks autorów

eISSN:	2719-9630
ISSN:	0138-0370